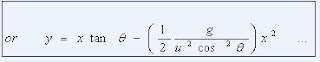- We know how to calculate the kinetic energy of moving objects -- isn't that enough? No. It turns out that many situations involving collisions do not obey the simple conservation of Mechanical Energy. Why not? Because it takes energy to bend, break, mutilate and deform objects, energy which disappears from the kinetic and gravitational potential energy.
- But a different quantity is conserved, even during collisions. The linear momentum of an object is defined as
p = (mass) * (velocity)
It is a vector quantity, and the total linear momentum of a bunch of objects will remain the same, before and after a collision.
- Momentum is connected to force by impulse, which is simply
impulse = (force) * (time)
if the force has a constant magnitude during its action. If the force changes with time, then one must integrate to find the impulse:/ impulse = | (force) dt/
- The Momentum-Impulse Theorem states that the change in momentum of an object is equal to the impulse exerted on it:
(change in momentum) = (impulse) p - p = (force) * (time) final initial m*v - m*v = (force) * (time) final initial
Impulse It is effect of force acting for a short
interval of time.
• According
to Newton’s 2nd law
• Rate
of change of momentum = Force applied.
• If
time period dt is increased
than Impact force decreases.
Why a cricketer lowers his
hands while catching a ball
• If
suppose a 0.16 kg cricket ball hits a bat at 100 km/hr and then comes off the
bat at 100 km/hr in 0.001 sec. The average force on ball is 8800 N which is
enough to lift 880 kg of mass off the ground.
That is why it hurts to get struck by ball. Therefore while catching
cricketer lowers his hands to increase time interval of change in momentum
thereby impact force reduces and the chances of any injury and avoiding reaction of hand that can drop the catch .